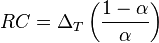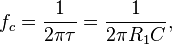"Xây dựng nền móng": 2009-10-11

Thứ Năm, 15 tháng 10, 2009

Thứ Tư, 14 tháng 10, 2009

Các mạch dao động cơ bản

Từ khóa:mạch dao động,khái niệm,thạch anh

1. Mạch tạo dao động

1.1 Mạch dao động hình Sin
Mạch tạo dao động hình Sin từ các linh kiện L – C hoặc từ thạch anh.

* Mạch dao động hình Sin dùng L – C

Mạch dao động hình Sin dùng L – C

Mach dao động trên có tụ C1 // L1 tạo thành mạch dao động L -C Để duy trì sự dao động này thì tín hiệu dao động được đưa vào chân B của Transistor, R1 là trở định thiên cho Transistor, R2 là trở gánh để lấy ra tín hiệu dao động ra , cuộn dây đấu từ chân E Transistor xuống mass có tác dụng lấy hồi tiếp để duy trì dao động. Tần số dao động của mạch phụ thuộc vào C1 và L1 theo công thức

f = 1 / 2.p.( L1.C1 )1/2

* Mạch dao động hình sin dùng thạch anh

Mạch tạo dao động bằng thạch anh

X1 : là thạch anh tạo dao động , tần số dao động được ghi trên thân của thach anh, khi thạch anh được cấp điện thì nó tự dao động ra sóng hình sin. Thạch anh thường có tần số dao động từ vài trăm KHz đến vài chục MHz.
Transistor Q1 khuyếch đại tín hiệu dao động từ thạch anh và cuối cùng tín hiệu được lấy ra ở chân C.
R1 vừa là điện trở cấp nguồn cho thạch anh vừa định thiên cho transistor Q1
R2 là trở ghánh tạo ra sụt áp để lấy ra tín hiệu .

1.2. Mạch dao động đa hài

Mạch dao động đa hài tạo xung vuông

* Bạn có thể tự lắp sơ đồ trên với các thông số như sau :

R1 = R4 = 1 KW
R2 = R3 = 100KW
C1 = C2 = 10µF/16V
Q1 = Q2 = đèn C828
Hai đèn Led
Nguồn Vcc là 6V DC

* Giải thích nguyên lý hoạt động

Khi cấp nguồn , giả sử đèn Q1 dẫn trước, áp Uc đèn Q1 giảm => thông qua C1 làm áp Ub đèn Q2 giảm => Q2 tắt => áp Uc đèn Q2 tăng => thông qua C2 làm áp Ub đèn Q1 tăng => xác lập trạng thái Q1 dẫn bão hoà và Q2 tắt , sau khoảng thời gian t , dòng nạp qua R3 vào tụ C1 khi điện áp này > 0,6V thì đèn Q2 dẫn => áp Uc đèn Q2 giảm => tiếp tục như vậy cho đến khi Q2 dẫn bão hoà và Q1 tắt, trạng thái lặp đi lặp lại và tạo thành dao động, chu kỳ dao động phụ thuộc vào C1, C2 và R2, R3.

IC tạo dao động XX555 ; XX có thể là TA hoặc LA v v …

Mạch dao động tạo xung bằng IC 555

Bạn hãy mua một IC họ 555 và tự lắp cho mình một mạch tạo dao động theo sơ đồ nguyên lý như trên.
Vcc cung cấp cho IC có thể sử dụng từ 4,5V đến 15V , đường mạch mầu đỏ là dương nguồn, mạch mầu đen dưới cùng là âm nguồn.
Tụ 103 (10nF) từ chân 5 xuống mass là cố định và bạn có thể bỏ qua ( không lắp cũng được )
Khi thay đổi các điện trở R1, R2 và giá trị tụ C1 bạn
sẽ thu được dao động có tần số và độ rộng xung theo ý muốn theo công
thức.

T = 0.7 × (R1 + 2R2) × C1 và f =	1.4
	(R1 + 2R2) × C1

T = Thời gian của một chu kỳ toàn phần tính bằng (s)
f = Tần số dao động tính bằng (Hz)
R1 = Điện trở tính bằng ohm (W )
R2 = Điện trở tính bằng ohm ( W )
C1 = Tụ điện tính bằng Fara ( W )

T = Tm + Ts
T : chu kỳ toàn phần
Tm = 0,7 x ( R1 + R2 ) x C1 Tm : thời gian điện mức cao
Ts = 0,7 x R2 x C1
Ts : thời gian điện mức thấp

Chu kỳ toàn phần T bao gồm thời gian có điện mức cao Tm và thời gian có điện mức thấp Ts

Từ các công thức trên ta có thể tạo ra một dao động xung vuông có độ rộng Tm và Ts bất kỳ.
Sau khi đã tạo ra xung có Tm và Ts ta có T = Tm + Ts và f = 1/ T

* Thí dụ bạn thiết kế mạch tạo xung dùng 555 có Tm = 0,1s , Ts = 1s

Với trị số linh kiện

C1 = 10µF = 10 x 10-6 = 10-5 F
R1 = R2 = 100K = 100 x 103 ohm

Tính Ts và Tm = ? Tính tần số f = ?

Ta có Ts = 0,7 x R2 x C1 = 0,7 x 100.103 x 10-5 = 0,7 s
Tm = 0,7 x ( R1 + R2 ) x C1 = 0,7 x 200.103 x 105 = 1,4 s
=> T = Tm + Ts = 1,4s + 0,7s = 2,1s
=> f =1 / T = 1/2,1 ~ 0,5 Hz

Mạch dao động nghẹt ( Blocking OSC )

Mạh dao động nghẹt có nguyên tắc hoạt động khá đơn giản, mạch
được sử dụng rộng rãi trong các bộ nguồn xung ( switching ), mạch có cấu tạo như sau :

Mạch dao động nghẹt

Mạch dao động nghẹt bao gồm

Biến áp : Gồm cuộn sơ cấp 1-2 và cuộn hồi tiếp 3-4, cuộn thứ cấp 5-6
Transistor Q tham gia dao động và đóng vai trò là đèn công xuất ngắt mở tạo ra dòng điện biến thiên qua cuộn sơ cấp.
Trở định thiên R1 ( là điện trở mồi )
R2, C2 là điện trở và tụ điện hồi tiếp

Có hai kiểu mắc hồi tiếp là hồi tiếp dương và hồi tiếp âm, ta xét cấu tạo và nguyên tắc hoạt động của từng mạch.

* Mạch dao động nghẹt hồi tiếp âm

Mạch hồi tiếp âm có cuộn hồi tiếp 3-4 quấn ngược chiều với cuộn sơ cấp 1-2 , và điện trở mồi R1 có trị số nhỏ khoảng 100K , mạch thường được sử dụng trong các bộ nguồn công xuất nhỏ khoảng 20W trở xuống
Nguyên tắc hoạt động : Khi cấp nguồn, dòng định thiên qua R1 kích cho đèn Q1 dẫn khá mạnh, dòng qua cuộn sơ cấp 1-2 tăng nhanh tạo ra từ trường biến thiên => cảm ứng sang cuộn hồi
tiếp, chiều âm của cuộn hồi tiếp được đưa về chân B đèn Q thông qua R2, C2 làm điện áp chân B đèn Q giảm < 0v =""> đèn Q lập tức chuyển sang trạng thái ngắt, sau khoảng thời gian t dòng điện qua R1 nạp vào tụ C2 làm áp chân B đèn Q tăng => đèn Q dẫn lặp lại chu kỳ thứ hai => tạo thành dao động .
Mạch dao động nghẹt hồi tiếp âm có ưu điểm là dao động nhanh, nhưng có nhược điểm dễ bị xốc điện làm hỏng đèn Q do đó mạch thường không sử dụng trong các bộ nguồn công suất lớn.

* Mạch dao động nghẹt hồi tiếp dương

Mạch dao động nghẹt hồi tiếp dương có cuộn hồi tiếp 3-4 quấn thuận chiều với cuộn sơ cấp 1-2, điện trở mồi R1 có trị số lớn khoảng 470K
Vì R1 có trị số lớn, lên dòng định thiên qua R1 ban đầu nhỏ => đèn Q dẫn tăng dần => sinh ra từ trường biến thiên cảm ứng lên cuộn hồi tiếp => điện áp hồi tiếp lấy chiều dương hồi tiếp qua R2, C2 làm đèn Q dẫn tăng => và tiếp tục cho đến khi đèn Q dẫn bão hoà, Khi đèn Q dẫn bão hoà, dòng điện qua cuộn 1-2 không đổi => mất điện áp hồi tiếp => áp chân B đèn Q giảm nhanh và đèn Q lập tức chuyển sang trạng thái ngắt, chu kỳ thứ hai lặp lại như trạng thái ban đầu và tạo thành dao động.
Mạch này có ưu điểm là rất an toàn dao động từ từ không bị xốc điện, và được sử dụng trong các mạch nguồn công xuất lớn như nguồn Ti vi mầu.

Sưu tầm: Lê viết vĩnh

Band-stop filter

A generic ideal band-stop filter, showing both positive and negative angular frequencies

In signal processing, a band-stop filter or band-rejection filter is a filter that passes most frequencies unaltered, but attenuates those in a specific range to very low levels. It is the opposite of a band-pass filter. A notch filter is a band-stop filter with a narrow stopband (high Q factor). Notch filters are used in live sound reproduction (Public Address systems, also known as PA systems) and in instrument amplifier (especially amplifiers or preamplifiers for acoustic instruments such as acoustic guitar, mandolin, bass instrument amplifier, etc.) to reduce or prevent feedback, while having little noticeable effect on the rest of the frequency spectrum. Other names include 'band limit filter', 'T-notch filter', 'band-elimination filter', and 'band-reject filter'.

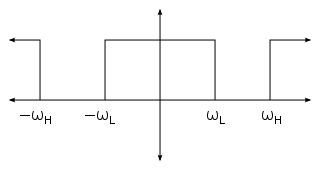
Typically, the width of the stopband is less than 1 to 2 decades (that is, the highest frequency attenuated is less than 10 to 100 times the lowest frequency attenuated). In the audio band, a notch filter uses high and low frequencies that may be only semitones apart.

Audio example 1: Anti-hum filter

Low Freq: 59 Hz
High Freq: 61 Hz

This means that the filter passes all frequencies, except for the range of 59–61 Hz. This would be used to filter out the mains hum from a 60 Hz power line, though its higher harmonics could still be present. The common European version of the filter would have a 49–51 Hz range.

Audio example 2: Anti-presence filter

Low Freq: 1 kHz
High Freq: 4 kHz

RF example 1: Non-linearities of power amplifiers For instance, when measuring non-linearities of power amplifiers a very narrow notch filter could be very useful to avoid the carrier so maximum input power of e.g. a spectrum analyser used to detect spurious content will not be exceeded.

Low-pass filter

A low-pass filter is a filter that passes low-frequency signals but attenuates (reduces the amplitude of) signals with frequencies higher than the cutoff frequency. The actual amount of attenuation for each frequency varies from filter to filter. It is sometimes called a high-cut filter, or treble cut filter when used in audio applications. A low-pass filter is the opposite of a high-pass filter, and a band-pass filter is a combination of a low-pass and a high-pass.

The concept of a low-pass filter exists in many different forms, including electronic circuits (like a hiss filter used in audio), digital algorithms for smoothing sets of data, acoustic barriers, blurring of images, and so on. Low-pass filters play the same role in signal processing that moving averages do in some other fields, such as finance; both tools provide a smoother form of a signal which removes the short-term oscillations, leaving only the long-term trend.

[hide]

[edit] Examples of low-pass filters

Figure 1: A low-pass electronic filter realized by an RC circuit

Figure 1 shows a low-pass RC filter for voltage signals, discussed in more detail below. Signal V_out contains frequencies from the input signal, with high frequencies attenuated, but with little attenuation below the cutoff frequency of the filter determined by its RC time constant. For current signals, a similar circuit using a resistor and capacitor in parallel works the same way. See current divider.

[edit] Acoustic

A stiff physical barrier tends to reflect higher sound frequencies, and so acts as a low-pass filter for transmitting sound. When music is playing in another room, the low notes are easily heard, while the high notes are attenuated.

[edit] Electronic

Electronic low-pass filters are used to drive subwoofers and other types of loudspeakers, to block high pitches that they can't efficiently broadcast.

Radio transmitters use low-pass filters to block harmonic emissions which might cause interference with other communications.

The tone knob found on many electric guitars is a low-pass filter used to reduce the amount of treble in the sound.

An integrator is another example of a low-pass filter.

DSL splitters use low-pass and high-pass filters to separate DSL and POTS signals sharing the same pair of wires.

Low-pass filters also play a significant role in the sculpting of sound for electronic music as created by analogue synthesisers. See subtractive synthesis.

[edit] Ideal and real filters

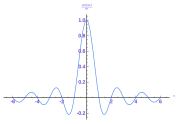
The sinc function, the impulse response of an ideal low-pass filter.

An ideal low-pass filter completely eliminates all frequencies above the cutoff frequency while passing those below unchanged: its frequency response is a rectangular function, and is a brick-wall filter. The transition region present in practical filters does not exist in an ideal filter. An ideal low-pass filter can be realized mathematically (theoretically) by multiplying a signal by the rectangular function in the frequency domain or, equivalently, convolution with its impulse response, a sinc function, in the time domain.

However, the ideal filter is impossible to realize without also having signals of infinite extent, and so generally needs to be approximated for real ongoing signals, because the sinc function's support region extends to all past and future times. The filter would therefore need to have infinite delay, or knowledge of the infinite future and past, in order to perform the convolution. It is effectively realizable for pre-recorded digital signals by assuming extensions of zero into the past and future, or more typically by making the signal repetitive and using Fourier analysis.

Real filters for real-time applications approximate the ideal filter by truncating and windowing the infinite impulse response to make a finite impulse response; applying that filter requires delaying the signal for a moderate period of time, allowing the computation to "see" a little bit into the future. This delay is manifested as phase shift. Greater accuracy in approximation requires a longer delay.

An ideal low-pass filter results in ringing artifacts via the Gibbs phenomenon. These can be reduced or worsened by choice of windowing function, and the design and choice of real filters involves understanding and minimizing these artifacts. For example, "simple truncation [of sinc] causes severe ringing artifacts," in signal reconstruction, and to reduce these artifacts one uses window functions "which drop off more smoothly at the edges."^[1]

The Whittaker–Shannon interpolation formula describes how to use a perfect low-pass filter to reconstruct a continuous signal from a sampled digital signal. Real digital-to-analog converters use real filter approximations.

[edit] Continuous-time low-pass filters

The gain-magnitude frequency response of a first-order (one-pole) low-pass filter. Power gain is shown in decibels (i.e., a 3 dB decline reflects an additional half-power attenuation). Angular frequency is shown on a logarithmic scale in units of radians per second.

There are a great many different types of filter circuits, with different responses to changing frequency. The frequency response of a filter is generally represented using a Bode plot, and the filter is characterized by its cutoff frequency and rate of frequency rolloff. In all cases, at the cutoff frequency, the filter attenuates the input power by half or 3 dB. So the order of the filter determines the amount of additional attenuation for frequencies higher than the cutoff frequency.

A first-order filter, for example, will reduce the signal amplitude by half (so power reduces by 6 dB) every time the frequency doubles (goes up one octave); more precisely, the power rolloff approaches 20 dB per decade in the limit of high frequency. The magnitude Bode plot for a first-order filter looks like a horizontal line below the cutoff frequency, and a diagonal line above the cutoff frequency. There is also a "knee curve" at the boundary between the two, which smoothly transitions between the two straight line regions. If the transfer function of a first-order low-pass filter has a zero as well as a pole, the Bode plot will flatten out again, at some maximum attenuation of high frequencies; such an effect is caused for example by a little bit of the input leaking around the one-pole filter; this one-pole–one-zero filter is still a first-order low-pass. See Pole–zero plot and RC circuit.

A second-order filter attenuates higher frequencies more steeply. The Bode plot for this type of filter resembles that of a first-order filter, except that it falls off more quickly. For example, a second-order Butterworth filter will reduce the signal amplitude to one fourth its original level every time the frequency doubles (so power decreases by 12 dB per octave, or 40 dB per decade). Other all-pole second-order filters may roll off at different rates initially depending on their Q factor, but approach the same final rate of 12 dB per octave; as with the first-order filters, zeroes in the transfer function can change the high-frequency asymptote. See RLC circuit.

Third- and higher-order filters are defined similarly. In general, the final rate of power rolloff for an order- $n$ all-pole filter is $6 n$ dB per octave (i.e., $20 n$ dB per decade).

On any Butterworth filter, if one extends the horizontal line to the right and the diagonal line to the upper-left (the asymptotes of the function), they will intersect at exactly the "cutoff frequency". The frequency response at the cutoff frequency in a first-order filter is 3 dB below the horizontal line. The various types of filters – Butterworth filter, Chebyshev filter, Bessel filter, etc. – all have different-looking "knee curves". Many second-order filters are designed to have "peaking" or resonance, causing their frequency response at the cutoff frequency to be above the horizontal line. See electronic filter for other types.

The meanings of 'low' and 'high' – that is, the cutoff frequency – depend on the characteristics of the filter. The term "low-pass filter" merely refers to the shape of the filter's response; a high-pass filter could be built that cuts off at a lower frequency than any low-pass filter – it is their responses that set them apart. Electronic circuits can be devised for any desired frequency range, right up through microwave frequencies (above 1 GHz) and higher.

[edit] Laplace notation

Continuous-time filters can also be described in terms of the Laplace transform of their impulse response in a way that allows all of the characteristics of the filter to be easily analyzed by considering the pattern of poles and zeros of the Laplace transform in the complex plane (in discrete time, one can similarly consider the Z-transform of the impulse response).

For example, a first-order low-pass filter can be described in Laplace notation as

$\frac{\text{Output}}{\text{Input}} = K \frac{1}{1 + s \tau}$

where s is the Laplace transform variable, τ is the filter time constant, and K is the filter passband gain.

[edit] Electronic low-pass filters

[edit] Passive electronic realization

Passive, first order low-pass RC filter

One simple electrical circuit that will serve as a low-pass filter consists of a resistor in series with a load, and a capacitor in parallel with the load. The capacitor exhibits reactance, and blocks low-frequency signals, causing them to go through the load instead. At higher frequencies the reactance drops, and the capacitor effectively functions as a short circuit. The combination of resistance and capacitance gives you the time constant of the filter $τ = R C$ (represented by the Greek letter tau). The break frequency, also called the turnover frequency or cutoff frequency (in hertz), is determined by the time constant:

$f_\mathrm{c} = {1 \over 2 \pi \tau } = {1 \over 2 \pi R C}$

or equivalently (in radians per second):

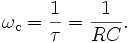
$\omega_\mathrm{c} = {1 \over \tau} = { 1 \over R C}.$

One way to understand this circuit is to focus on the time the capacitor takes to charge. It takes time to charge or discharge the capacitor through that resistor:

At low frequencies, there is plenty of time for the capacitor to charge up to practically the same voltage as the input voltage.
At high frequencies, the capacitor only has time to charge up a small amount before the input switches direction. The output goes up and down only a small fraction of the amount the input goes up and down. At double the frequency, there's only time for it to charge up half the amount.

Another way to understand this circuit is with the idea of reactance at a particular frequency:

Since DC cannot flow through the capacitor, DC input must "flow out" the path marked $V out$ (analogous to removing the capacitor).
Since AC flows very well through the capacitor — almost as well as it flows through solid wire — AC input "flows out" through the capacitor, effectively short circuiting to ground (analogous to replacing the capacitor with just a wire).

It should be noted that the capacitor is not an "on/off" object (like the block or pass fluidic explanation above). The capacitor will variably act between these two extremes. It is the Bode plot and frequency response that show this variability.

[edit] Active electronic realization

An active low-pass filter

Another type of electrical circuit is an active low-pass filter.

In the operational amplifier circuit shown in the figure, the cutoff frequency (in hertz) is defined as:

$f_{\text{c}} = \frac{1}{2 \pi R_2 C}$

or equivalently (in radians per second):

$\omega_{\text{c}} = \frac{1}{R_2 C}$

The gain in the passband is 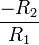$\frac{-R_2}{R_1}$ , and the stopband drops off at −6 dB per octave as it is a first-order filter.

Sometimes, a simple gain amplifier (as opposed to the very-high-gain operation amplifier) is turned into a low-pass filter by simply adding a feedback capacitor C. This feedback decreases the frequency response at high frequencies via the Miller effect, and helps to avoid oscillation in the amplifier. For example, an audio amplifier can be made into a low-pass filter with cutoff frequency 100 kHz to reduce gain at frequencies which would otherwise oscillate. Since the audio band (what we can hear) only goes up to 20 kHz or so, the frequencies of interest fall entirely in the passband, and the amplifier behaves the same way as far as audio is concerned.

[edit] Discrete-time realization

For another method of conversion from continuous- to discrete-time, see Bilinear transform.

The effect of a low-pass filter can be simulated on a computer by analyzing its behavior in the time domain, and then discretizing the model.

A simple low-pass RC filter

From the circuit diagram to the right, according to Kirchoff's Laws and the definition of capacitance:

$\begin{cases} v_{\text{in}}(t) - v_{\text{out}}(t) = R \, i(t)&\text{(V)}\\ Q_c(t) = C \, v_{\text{out}}(t)&\text{(Q)}\\ i(t) = \frac{\operatorname{d} Q_c}{\operatorname{d} t} &\text{(I)} \end{cases}$

where $Q c (t)$ is the charge stored in the capacitor at time $t$ . Substituting Equation (Q) into Equation (I) gives $i(t) = C \frac{\operatorname{d}v_{\text{out}}}{\operatorname{d}t}$ , which can be substituted into Equation (V) so that:

$v_{\text{in}}(t) - v_{\text{out}}(t) = RC \frac{\operatorname{d}v_{\text{out}}}{\operatorname{d}t}\,$

This equation can be discretized. For simplicity, assume that samples of the input and output are taken at evenly-spaced points in time separated by $Δ T$ time. Let the samples of $v in$ be represented by the sequence $(x_1, x_2, \ldots, x_n)$ , and let $v out$ be represented by the sequence $(y_1, y_2, \ldots, y_n)$ which correspond to the same points in time. Making these substitutions:

$x_i - y_i = RC \, \frac{y_{i}-y_{i-1}}{\Delta_T}\,$

And rearranging terms gives the recurrence relation

$y_i = \overbrace{x_i \left( \frac{\Delta_T}{RC + \Delta_T} \right)}^{\text{Input contribution}} + \overbrace{y_{i-1} \left( \frac{RC}{RC + \Delta_T} \right)}^{\text{Inertia from previous output}}$

That is, this discrete-time implementation of a simple RC low-pass filter is the exponentially-weighted moving average

$y_i = \alpha x_i + (1 - \alpha) y_{i-1} \qquad \text{where} \qquad \alpha \triangleq \frac{\Delta_T}{RC + \Delta_T}\,$

By definition, the smoothing factor $0 \leq \alpha \leq 1$ . The expression for $α$ yields the equivalent time constant $R C$ in terms of the sampling period $Δ T$ and smoothing factor $α$ :

$RC = \Delta_T \left( \frac{1 - \alpha}{\alpha} \right)$

If $α = 0.5$ , then the $R C$ time constant equal to the sampling period. If 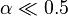$\alpha \ll 0.5$ , then $R C$ is significantly larger than the sampling interval, and $\Delta_T \approx \alpha RC$ .

[edit] Algorithmic implementation

The filter recurrence relation provides a way to determine the output samples in terms of the input samples and the preceding output. The following pseudocode algorithm will simulate the effect of a low-pass filter on a series of digital samples:

 // Return RC low-pass filter output samples, given input samples,
// time interval dt, and time constant RC
function lowpass(real[0..n] x, real dt, real RC)
  var real[0..n] y
  var real α := dt / (RC + dt)
  y[0] := x[0]
  for i from 1 to n
      y[i] := α * x[i] + (1-α) * y[i-1]   
  return y

The loop which calculates each of the $n$ outputs can be refactored into the equivalent:

   for i from 1 to n
      y[i] := y[i-1] + α * (x[i] - y[i-1])

That is, the change from one filter output to the next is proportional to the difference between the previous output and the next input. This exponential smoothing property matches the exponential decay seen in the continuous-time system. As expected, as the time constant $R C$ increases, the discrete-time smoothing parameter $α$ decreases, and the output samples $(y_1,y_2,\ldots,y_n)$ respond more slowly to a change in the input samples $(x_1,x_2,\ldots,x_n)$ – the system will have more inertia.

High-pass filter

A high-pass filter is an LTI filter that passes high frequencies well but attenuates (i.e., reduces the amplitude of) frequencies lower than the cutoff frequency. The actual amount of attenuation for each frequency is a design parameter of the filter. It is sometimes called a low-cut filter; the terms bass-cut filter or rumble filter are also used in audio applications.^{[citation needed]}

[hide]

[edit] First-order continuous-time implementation

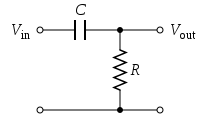
Figure 1: A passive, analog, first-order high-pass filter, realized by an RC circuit

The simple first-order electronic high-pass filter shown in Figure 1 is implemented by placing an input voltage across the series combination of a capacitor and a resistor and using the voltage across the resistor as an output. The product of the resistance and capacitance (R×C) is the time constant (τ); it is inversely proportional to the cutoff frequency f_c, at which the output power is half (−3 dB) the input power. That is,

$f_c = \frac{1}{2 \pi \tau} = \frac{1}{2 \pi R C},\,$

where f_c is in Hertz, τ is in seconds, R is in Ohms, and C is in Farads.

Figure 2: An active high-pass filter

Figure 2 shows an active electronic implementation of a first-order high-pass filter using an operational amplifier. In this case, the filter has a passband gain of -R₂/R₁ and has a corner frequency of

$f_c = \frac{1}{2 \pi \tau} = \frac{1}{2 \pi R_1 C},\,$

Because this filter is active, it may have non-unity passband gain. That is, high-frequency signals are inverted and amplified by R₂/R₁.

[edit] Discrete-time realization

For another method of conversion from continuous- to discrete-time, see Bilinear transform.

Discrete-time high-pass filters can also be designed. Discrete-time filter design is beyond the scope of this article; however, a simple example comes from the conversion of the continuous-time high-pass filter above to a discrete-time realization. That is, the continuous-time behavior can be discretized.

From the circuit in Figure 1 above, according to Kirchoff's Laws and the definition of capacitance:

$\begin{cases} V_{\text{out}}(t) = I(t)\, R &\text{(V)}\\ Q_c(t) = C \, \left( V_{\text{in}}(t) - V_{\text{out}}(t) \right) &\text{(Q)}\\ I(t) = \frac{\operatorname{d} Q_c}{\operatorname{d} t} &\text{(I)} \end{cases}$

where $Q c (t)$ is the charge stored in the capacitor at time $t$ . Substituting Equation (Q) into Equation (I) and then Equation (I) into Equation (V) gives:

$V_{\text{out}}(t) = \overbrace{C \, \left( \frac{\operatorname{d} V_{\text{in}}}{\operatorname{d}t} - \frac{\operatorname{d} V_{\text{out}}}{\operatorname{d}t} \right)}^{I(t)} \, R = R C \, \left( \frac{ \operatorname{d} V_{\text{in}}}{\operatorname{d}t} - \frac{\operatorname{d} V_{\text{out}}}{\operatorname{d}t} \right)$

This equation can be discretized. For simplicity, assume that samples of the input and output are taken at evenly-spaced points in time separated by $Δ T$ time. Let the samples of $V in$ be represented by the sequence $(x_1, x_2, \ldots, x_n)$ , and let $V out$ be represented by the sequence $(y_1, y_2, \ldots, y_n)$ which correspond to the same points in time. Making these substitutions:

$y_i = R C \, \left( \frac{x_i - x_{i-1}}{\Delta_T} - \frac{y_i - y_{i-1}}{\Delta_T} \right)$

And rearranging terms gives the recurrence relation

$y_i = \overbrace{\frac{RC}{RC + \Delta_T} y_{i-1}}^{\text{Decaying contribution from prior inputs}} + \overbrace{\frac{RC}{RC + \Delta_T} \left( x_i - x_{i-1} \right)}^{\text{Contribution from change in input}}$

That is, this discrete-time implementation of a simple continuous-time RC high-pass filter is

$y_i = \alpha y_{i-1} + \alpha (x_{i} - x_{i-1}) \qquad \text{where} \qquad \alpha \triangleq \frac{RC}{RC + \Delta_T}$

By definition, $0 \leq \alpha \leq 1$ . The expression for parameter $α$ yields the equivalent time constant $R C$ in terms of the sampling period $Δ T$ and $α$ :

$RC = \Delta_T \left( \frac{\alpha}{1 - \alpha} \right)$

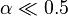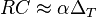If $α = 0.5$ , then the $R C$ time constant equal to the sampling period. If $\alpha \ll 0.5$ , then $R C$ is significantly smaller than the sampling interval, and $RC \approx \alpha \Delta_T$ .

[edit] Algorithmic implementation

 // Return RC high-pass filter output samples, given input samples,
// time interval dt, and time constant RC
function highpass(real[0..n] x, real dt, real RC)
  var real[0..n] y
  var real α := RC / (RC + dt)
  y[0] := x[0]
  for i from 1 to n
    y[i] := α * y[i-1] + α * (x[i] - x[i-1])
  return y

The loop which calculates each of the $n$ outputs can be refactored into the equivalent:

   for i from 1 to n
    y[i] := α * (y[i-1] + x[i] - x[i-1])

However, the earlier form shows how the parameter α changes the impact of the prior output y[i-1] and current change in input (x[i] - x[i-1]). In particular,

A large α implies that the output will decay very slowly but will also be strongly influenced by even small changes in input. By the relationship between parameter α and time constant $R C$ above, a large α corresponds to a large $R C$ and therefore a low corner frequency of the filter. Hence, this case corresponds to a high-pass filter with a very narrow stop band. Because it is excited by small changes and tends to hold its prior output values for a long time, it can pass relatively low frequencies. However, a constant input (i.e., an input with (x[i] - x[i-1])=0) will always decay to zero, as would be expected with a high-pass filter with a large $R C$ .
A small α implies that the output will decay quickly and will require large changes in the input (i.e., (x[i] - x[i-1]) is large) to cause the output to change much. By the relationship between parameter α and time constant $R C$ above, a small α corresponds to a small $R C$ and therefore a high corner frequency of the filter. Hence, this case corresponds to a high-pass filter with a very wide stop band. Because it requires large (i.e., fast) changes and tends to quickly forget its prior output values, it can only pass relatively high frequencies, as would be expected with a high-pass filter with a small $R C$ .

[edit] Applications

Such a filter could be used as part of an audio crossover to direct high frequencies to a tweeter while blocking bass signals which could interfere with, or damage, the speaker. When such a filter is built into a loudspeaker cabinet it is normally a passive filter that also includes a low-pass filter for the woofer and so often employs both a capacitor and inductor (although very simple high-pass filters for tweeters can consist of a series capacitor and nothing else). An alternative, which provides good quality sound without inductors (which are prone to parasitic coupling, are expensive, and may have significant internal resistance) is to employ bi-amplification with active RC filters with separate power amplifiers for each loudspeaker making an active crossover.^{[citation needed]}

Rumble filters are high-pass filters applied to the removal of unwanted sounds below or near to the lower end of the audible range. For example, noises (e.g., footsteps, or motor noises from record players and tape decks) may be removed because they are undesired or may overload the RIAA equalization circuit of the preamp.^{[citation needed]}

High-pass and low-pass filters are also used in digital image processing to perform transformations in the spatial frequency domain.^{[citation needed]}

High-pass filters are also used for AC coupling at the input and output of amplifiers.^{[citation needed]}

"Xây dựng nền móng"

Thứ Năm, 15 tháng 10, 2009

Chứng chỉ quốc tế .

Thứ Tư, 14 tháng 10, 2009

Các mạch dao động cơ bản

Band-stop filter

Low-pass filter

Contents

[edit] Examples of low-pass filters

[edit] Acoustic

[edit] Electronic

[edit] Ideal and real filters

[edit] Continuous-time low-pass filters

[edit] Laplace notation

[edit] Electronic low-pass filters

[edit] Passive electronic realization

[edit] Active electronic realization

[edit] Discrete-time realization

[edit] Algorithmic implementation

High-pass filter

Contents

[edit] First-order continuous-time implementation

[edit] Discrete-time realization

[edit] Algorithmic implementation

[edit] Applications

Vi tính - VnExpress.net

Cười - VnExpress.net

Facebook Badge

Profile of Viết Vĩnh

Lưu trữ Blog của Viết Vĩnh

Link liên kết cũng khá hay đây bà con!!!!

Tìm kiếm Blog này

Xe khủng long

Phần mềm nghe nhạc.

"Xây dựng nền móng"

Thứ Năm, 15 tháng 10, 2009

Chứng chỉ quốc tế .

Thứ Tư, 14 tháng 10, 2009

Các mạch dao động cơ bản

Band-stop filter

Low-pass filter

Contents

[edit] Examples of low-pass filters

[edit] Acoustic

[edit] Electronic

[edit] Ideal and real filters

[edit] Continuous-time low-pass filters

[edit] Laplace notation

[edit] Electronic low-pass filters

[edit] Passive electronic realization

[edit] Active electronic realization

[edit] Discrete-time realization

[edit] Algorithmic implementation

High-pass filter

Contents

[edit] First-order continuous-time implementation

[edit] Discrete-time realization

[edit] Algorithmic implementation

[edit] Applications

Vi tính - VnExpress.net

Cười - VnExpress.net

Facebook Badge

Profile of Viết Vĩnh

Lưu trữ Blog của Viết Vĩnh

Đăng ký

Link liên kết cũng khá hay đây bà con!!!!

Tìm kiếm Blog này

Xe khủng long

Phần mềm nghe nhạc.